Limit Of E 1 X As X Approaches 0

Lim x 2 2x 3 x 2 2x 3 as x 3. Take the limit of each term. As the values approach from the left the function values increase without bound.

lista patrate perfecte pana la 10000 lista patrate perfecte pana la 1000 lista pensiuni vouchere vacanta 2020 lista locuri parcare sector 3 2020 lista lui schindler film online lista cladiri risc seismic lista filme de dragoste lista contribuabili mijlocii 2017

Show Limit Does Not Exist As X Approaches Zero For E 1 X Youtube

Limit Of E 1 X As X 0 Physics Forums

Limit Of 1 X 1 X As X Approaches 0 Youtube

What Is The Limit Of 1 X As X Approaches 0 Quora

Limits Of Transendental Function E 1 X 1 E 1 X 1 As X Approaches Zero Test 1 Youtube

Prove The Limit As X Approaches 0 Of E X 1 X Youtube

Tap for more steps.

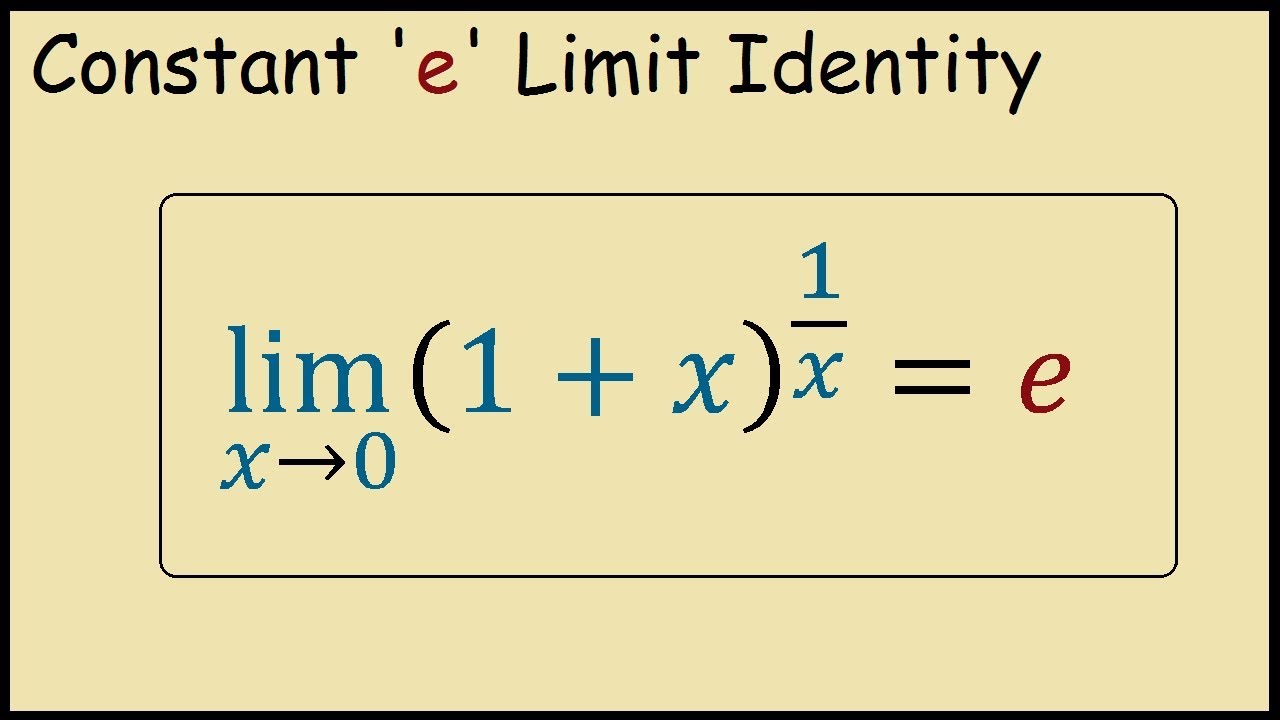
Limit of e 1 x as x approaches 0. Displaystyle large lim x to 0 normalsize dfrac e displaystyle normalsize x 1 x the limit of this special exponential function is equal to one. If c is positive b approaches infinity. Evaluate limit as x approaches 0 of e 4x 1 x. Limit 1 1 n n as n infinity.

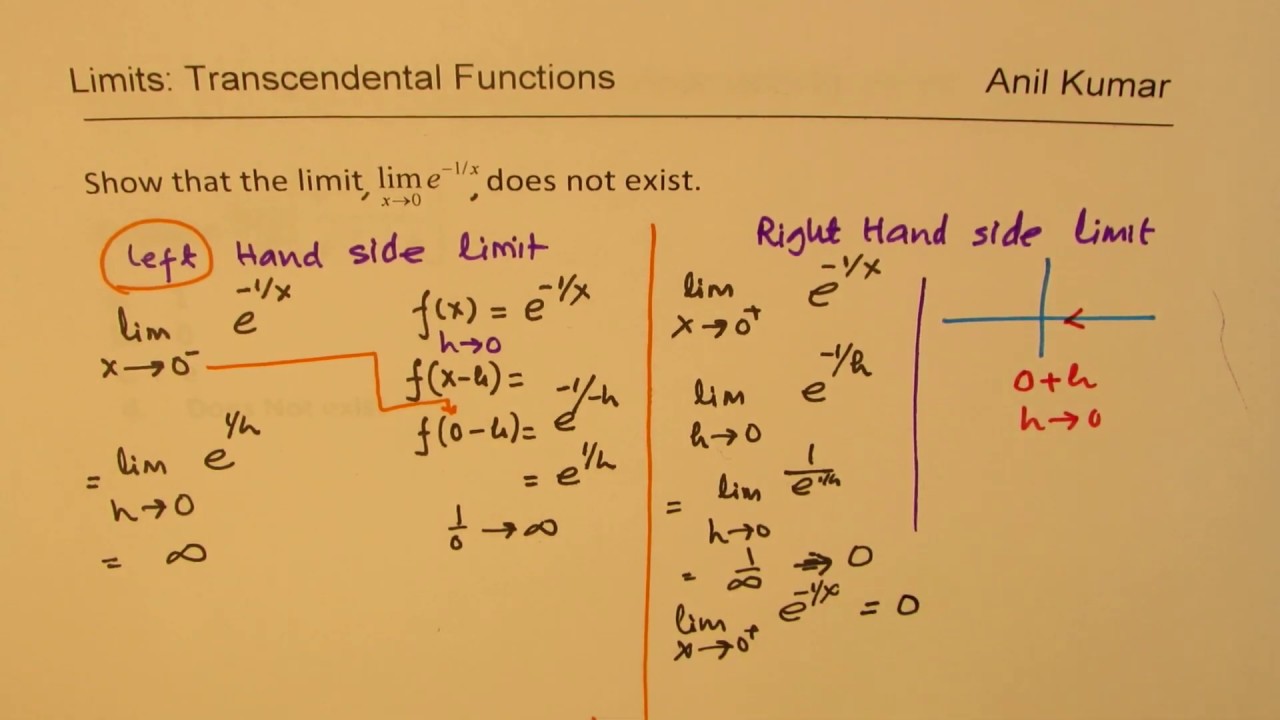
In this case if x 0 that means 1 0 which does not exist which means this function is discontinuous hence why we approach from the left of the right. Lim x x as. Tap for more steps. Split the limit using the sum of limits rule on the limit as approaches.

Next layer b e 1 x to follow our theme lets make this b e c. Or since it is the 1 x that bothers you let y 1 x. As x goes to 0 from the right 1 x goes to positive infinity so the problem becomes displaystyle displaystyle lim y to infty frac e y 1 e y 1. Evaluate limit as x approaches 0 of e 1 x consider the left sided limit.

For a directional limit use either the or sign or plain english such as left above right or below limit sin x x as x 0. Evaluate the limit of the numerator and the limit of the denominator. If c is negative b approaches 0. There are two sides to the limit.

For specifying a limit argument x and point of approach a type x a. Lim x h 5 x 5 h as h 0. The standard result of this limit of exponential function can be expressed in several ways in calculus.